


1. Choice of array, which is more sensitive to anisotropy
At study of anisotropic media with the help of azimuthal resistivity survey
prof. A.S.Semenov has recommended to
apply dipole equatorial array or any other non-linear array. Arrays with
electrodes' position not in one line (non-linear arrays) have higher
sensitivity to anisotropy in comparison with linear (or collinear)
arrays (Schlumberger, AM, AMN).
 The most simple example
of non-linear array is dipole equatorial (D) array. In attempt to connect
advantages D (T) and Schlumberger arrays and simultaneously to overcome
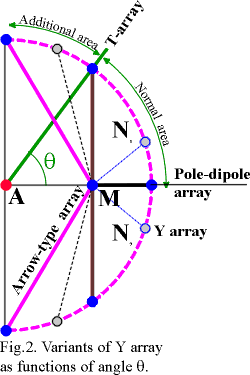
their lacks the generalized Y-array was invented (fig.2). As T - array
it has two MN lines
The most simple example
of non-linear array is dipole equatorial (D) array. In attempt to connect
advantages D (T) and Schlumberger arrays and simultaneously to overcome
their lacks the generalized Y-array was invented (fig.2). As T - array
it has two MN lines 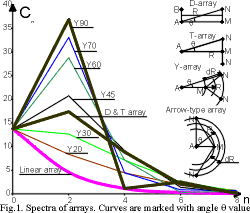 with
one common M electrode. These two MN lines go under equal angles from M
electrode position symmetrically from AM direction. But this array has
one important feature - two MN lines can influence at level of signal while
the radiuses' difference (between A and M, A and N), and on sensitivity
to an anisotropy depends on angle between AM and AN azimuths. Really, by
joining two MN "wings" we receive low sensitive pole-dipole array, and
by flinging them we receive T-array, which is more sensitive. It can be
shown with the help of spectra (fig.1). But it has appeared, that the wings
can be bent in additional area (fig.2), and in this case sensitivity to
an anisotropy continues to grow. On this way an arrow-type array (AT)
was revealed. Except higher sensitivity to an anisotropy, it has some interesting
and useful properties.
with
one common M electrode. These two MN lines go under equal angles from M
electrode position symmetrically from AM direction. But this array has
one important feature - two MN lines can influence at level of signal while
the radiuses' difference (between A and M, A and N), and on sensitivity
to an anisotropy depends on angle between AM and AN azimuths. Really, by
joining two MN "wings" we receive low sensitive pole-dipole array, and
by flinging them we receive T-array, which is more sensitive. It can be
shown with the help of spectra (fig.1). But it has appeared, that the wings
can be bent in additional area (fig.2), and in this case sensitivity to
an anisotropy continues to grow. On this way an arrow-type array (AT)
was revealed. Except higher sensitivity to an anisotropy, it has some interesting
and useful properties.
1. It was proved theoretically that the signals in both MN lines of
arrow-type array above anisotropic media are completely identical. For
T and Y arrays it is not so. This property above anisotropic media allows
to measure signal only in one MN line. The measurements in two MN lines
help to distinguish inhomogeneous medium from anisotropic one by taking
into account the difference of two signals.
 2. The spectrum of
arrow-type array appears very narrow, the harmonics after fourth one can
be neglected, and consequently for azimuthal survey the step in 45°
is sufficient. The step size is similar to linear array, but sensitivity
is higher, than for dipole array (fig.1.). That is because two MN lines
are at azimuths with 90° difference. The periodicity of the second
harmonic is 180°, i.e. its amplitude for the given array will be maximal,
whereas the periodicity of the fourth harmonic is 90° and its amplitude
for the given array will be equal to zero. Fourth, eight and some other
harmonics are exactly equal to zero, when the radiuses AM and AN are identical.
But the possibility of apparent resistivity calculation in this case disappears.
If we make these distances different, the fourth harmonic will not be zero,
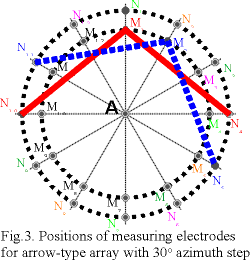
though also small. Arrow-type array is rather convenient for field survey.
The scheme of electrodes positions for an azimuth step in 30° is shown
in fig.3.
2. The spectrum of
arrow-type array appears very narrow, the harmonics after fourth one can
be neglected, and consequently for azimuthal survey the step in 45°
is sufficient. The step size is similar to linear array, but sensitivity
is higher, than for dipole array (fig.1.). That is because two MN lines
are at azimuths with 90° difference. The periodicity of the second
harmonic is 180°, i.e. its amplitude for the given array will be maximal,
whereas the periodicity of the fourth harmonic is 90° and its amplitude
for the given array will be equal to zero. Fourth, eight and some other
harmonics are exactly equal to zero, when the radiuses AM and AN are identical.
But the possibility of apparent resistivity calculation in this case disappears.
If we make these distances different, the fourth harmonic will not be zero,
though also small. Arrow-type array is rather convenient for field survey.
The scheme of electrodes positions for an azimuth step in 30° is shown
in fig.3.
119899, Russia, Moscow, Moscow State University, Geological
Faculty, Department of Geophysics
V.A. Shevnin, I.N. Modin
Tel. & fax: (7-095) 939 49 63
E-mail: sh@geophys.geol.msu.ru
Please feel free to send us
your comments.



 The most simple example
of non-linear array is dipole equatorial (D) array. In attempt to connect
advantages D (T) and Schlumberger arrays and simultaneously to overcome
their lacks the generalized Y-array was invented (fig.2). As T - array
it has two MN lines
The most simple example
of non-linear array is dipole equatorial (D) array. In attempt to connect
advantages D (T) and Schlumberger arrays and simultaneously to overcome
their lacks the generalized Y-array was invented (fig.2). As T - array
it has two MN lines  with
one common M electrode. These two MN lines go under equal angles from M
electrode position symmetrically from AM direction. But this array has
one important feature - two MN lines can influence at level of signal while
the radiuses' difference (between A and M, A and N), and on sensitivity
to an anisotropy depends on angle between AM and AN azimuths. Really, by
joining two MN "wings" we receive low sensitive pole-dipole array, and
by flinging them we receive T-array, which is more sensitive. It can be
shown with the help of spectra (fig.1). But it has appeared, that the wings
can be bent in additional area (fig.2), and in this case sensitivity to
an anisotropy continues to grow. On this way an arrow-type array (AT)
was revealed. Except higher sensitivity to an anisotropy, it has some interesting
and useful properties.
with
one common M electrode. These two MN lines go under equal angles from M
electrode position symmetrically from AM direction. But this array has
one important feature - two MN lines can influence at level of signal while
the radiuses' difference (between A and M, A and N), and on sensitivity
to an anisotropy depends on angle between AM and AN azimuths. Really, by
joining two MN "wings" we receive low sensitive pole-dipole array, and
by flinging them we receive T-array, which is more sensitive. It can be
shown with the help of spectra (fig.1). But it has appeared, that the wings
can be bent in additional area (fig.2), and in this case sensitivity to
an anisotropy continues to grow. On this way an arrow-type array (AT)
was revealed. Except higher sensitivity to an anisotropy, it has some interesting
and useful properties.
 2. The spectrum of
arrow-type array appears very narrow, the harmonics after fourth one can
be neglected, and consequently for azimuthal survey the step in 45°
is sufficient. The step size is similar to linear array, but sensitivity
is higher, than for dipole array (fig.1.). That is because two MN lines
are at azimuths with 90° difference. The periodicity of the second
harmonic is 180°, i.e. its amplitude for the given array will be maximal,
whereas the periodicity of the fourth harmonic is 90° and its amplitude
for the given array will be equal to zero. Fourth, eight and some other
harmonics are exactly equal to zero, when the radiuses AM and AN are identical.
But the possibility of apparent resistivity calculation in this case disappears.
If we make these distances different, the fourth harmonic will not be zero,
though also small. Arrow-type array is rather convenient for field survey.
The scheme of electrodes positions for an azimuth step in 30° is shown
in fig.3.
2. The spectrum of
arrow-type array appears very narrow, the harmonics after fourth one can
be neglected, and consequently for azimuthal survey the step in 45°
is sufficient. The step size is similar to linear array, but sensitivity
is higher, than for dipole array (fig.1.). That is because two MN lines
are at azimuths with 90° difference. The periodicity of the second
harmonic is 180°, i.e. its amplitude for the given array will be maximal,
whereas the periodicity of the fourth harmonic is 90° and its amplitude
for the given array will be equal to zero. Fourth, eight and some other
harmonics are exactly equal to zero, when the radiuses AM and AN are identical.
But the possibility of apparent resistivity calculation in this case disappears.
If we make these distances different, the fourth harmonic will not be zero,
though also small. Arrow-type array is rather convenient for field survey.
The scheme of electrodes positions for an azimuth step in 30° is shown
in fig.3.